
Electromagnetism, a fundamental branch of physics, encompasses the study of electric and magnetic fields and their interactions. It’s a fascinating subject that reveals the hidden forces governing much of the physical world. From the simple act of flipping a light switch to the intricate workings of our electronic devices, electromagnetism is omnipresent.
One useful resource for navigating these difficulties is exploring solved problems in electromagnetism, which provides practical examples and solutions, offering a clearer understanding of how to approach and solve various electromagnetic problems.
Table of Contents
Conceptual Understanding

Source: thoughtco.com
A primary challenge students face in electromagnetism is developing a deep conceptual understanding of the subject. Electromagnetic theory involves abstract concepts that can be difficult to visualize and comprehend. For instance, understanding how electric and magnetic fields interact and propagate through space requires a leap from tangible, everyday experiences to more abstract thinking.
Mathematical Rigor
The mathematical demands of electromagnetism are another significant hurdle. Electromagnetic theory is deeply rooted in calculus, differential equations, and vector calculus. Students often find the mathematical rigor daunting, particularly if they have not yet developed a strong foundation in these areas.
Vector calculus, for example, is integral to understanding and applying Maxwell’s equations. Students must be comfortable with operations such as gradient, divergence, and curl, and understand how these operations apply to physical fields. Additionally, solving problems often involves working with partial differential equations, which can be complex and require sophisticated techniques.
Problem-Solving Skills
One common issue is the tendency to focus on memorizing formulas without understanding when and how to apply them. Electromagnetic problems often require a multi-step approach, where students must first conceptualize the physical situation, set up the relevant equations, and then solve them using appropriate mathematical techniques. This process can be overwhelming, especially when the problems are complex and involve multiple variables and steps.
Experimental and Practical Aspects
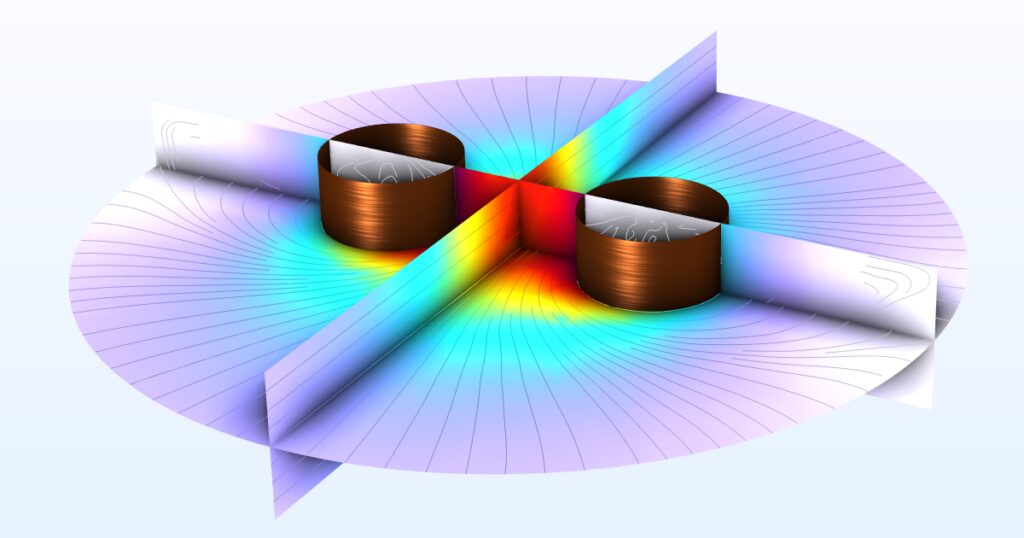
Source: comsol.com
Understanding the practical applications and experimental aspects of electromagnetism is crucial but challenging for students. Electromagnetic principles are not only theoretical; they have direct applications in various technologies and scientific experiments.
Students often struggle with designing and interpreting experiments related to electromagnetism. This includes setting up experiments to measure electric and magnetic fields, understanding the limitations of measurement techniques, and analyzing experimental data. The gap between theoretical knowledge and practical application can be significant, and bridging this gap requires hands-on experience and a thorough understanding of experimental methods.
Relativity and Electrodynamics
Electromagnetism is closely linked to the theory of relativity, particularly special relativity. This connection can be a significant challenge for students, as it requires integrating concepts from both fields.
Maxwell’s equations are invariant under Lorentz transformations, and understanding this invariance is crucial for a complete understanding of electromagnetism. Students must grapple with concepts such as time dilation, length contraction, and the relativity of simultaneity, and understand how these concepts affect electromagnetic phenomena.
Interdisciplinary Connections
Source: sharemylesson.com
Electromagnetism is a field with broad interdisciplinary connections, linking physics with other scientific and engineering disciplines. This interdisciplinary nature can be both an opportunity and a challenge for students.
In addition, electromagnetism is fundamental to electrical and electronic engineering. Understanding how electromagnetic principles are applied in designing and analyzing circuits, devices, and systems is essential for students pursuing careers in these fields. This requires not only a solid grasp of the theoretical principles but also the ability to apply them in practical engineering contexts.







